Dynamical systems are constructions that capture the evolution of mathematical structures over time. Modern research in the study of dynamical systems includes the exploration of questions of a purely theoretical nature, with deep connections to geometry, analysis, algebra, and number theory. Dynamical systems are also applicable across diverse fields and help in predicting behaviors and understanding complex patterns in natural and artificial systems, from epidemiology and population modeling to data science and machine learning.
Dynamical Systems
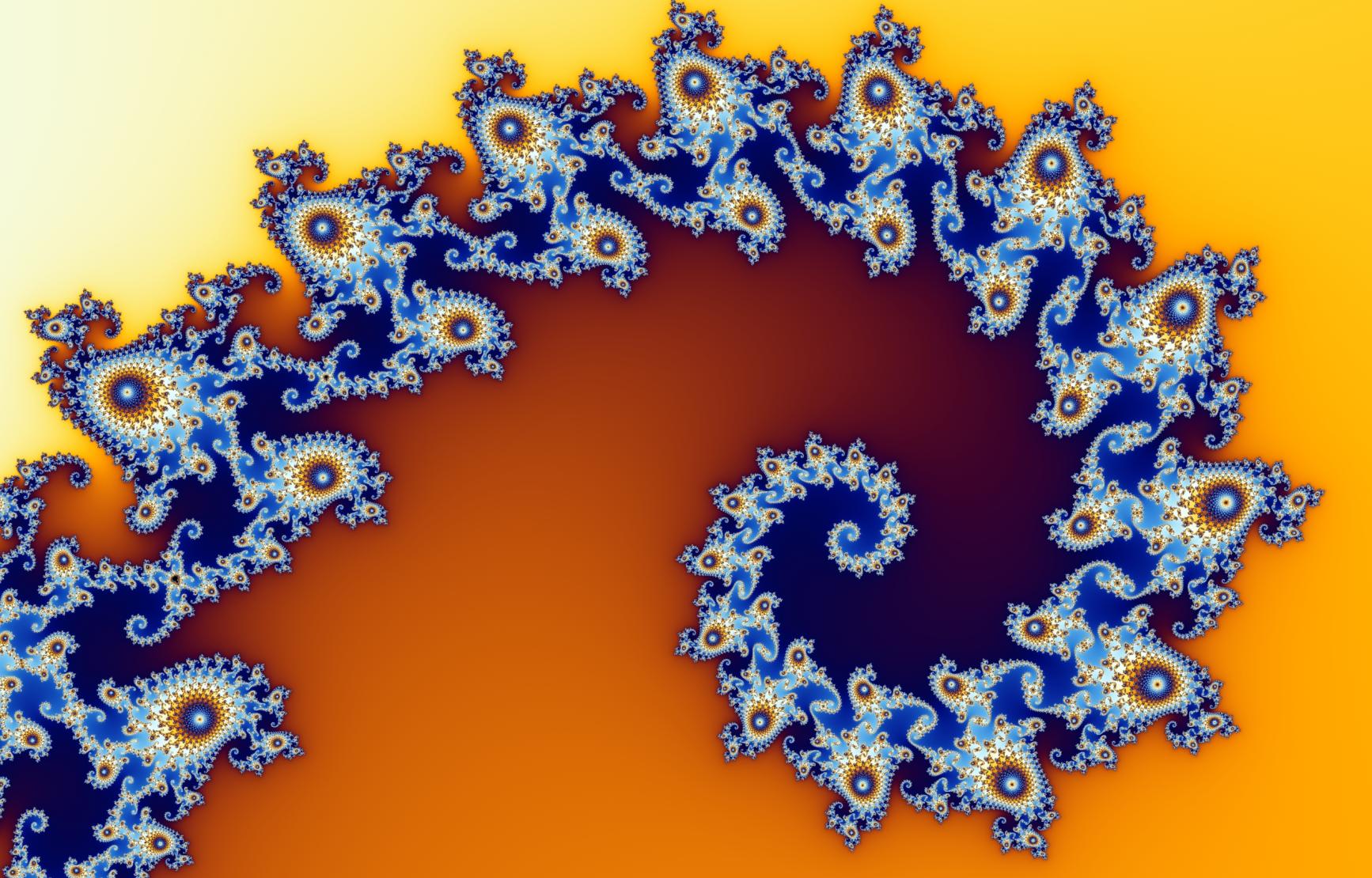
In dynamical systems, iteration of functions as simple as quadratic polynomials can produce stunning complexity. The Mandelbrot set is the collection of quadratic polynomial dynamical systems on the complex plane whose unique critical point has bounded forward orbit. This photo provides a close up view of part of the Mandelbrot set near a Misiurewicz point. When viewed as dynamical systems on a real interval, quadratic polynomials also provide foundational models for population dynamics in mathematical biology. (photo credit)















